Reliability Study of Notched Composite Laminates Under Uniaxial Loading Based on Continuum Damage Mechanics Approach

Abstract

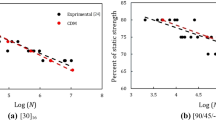
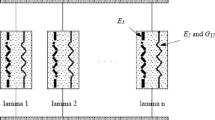
The probability of failure estimation of composite structures under applied loads is an inevitable requirement given the uncertainties related to the material properties, loads, and boundary conditions. The properties of composite materials have more scatter due to non-homogeneity and anisotropic characteristics, and manufacturing defects. In this paper, the reliability of S2-Glass/Epoxy laminate composite materials containing a central circular hole under static tensile load is presented. Failure in fiber-reinforced plastic structures, since individual faults in each ply cannot be traced, is a random process due to the scatter caused by the behavior of the material. According to the continuum damage mechanics (CDM) approach, a damage model proposed by Ladeveze is used to model the matrix cracking and fiber/matrix debonding and then, the material constitutive relationships are implemented in the ABAQUS software by the subroutine. The first-order reliability method (FORM) and second-order reliability method (SORM) have been used to analyze the system failure probability of the composite plates, and the failure functions and random variables have been obtained according to the CDM approach. Scatter in random parameters have been displayed to have a significant effect on damage development. Finally, using sensitivity analysis, sensitive and effective parameters in the reliability of laminate composite were introduced.

Appendix

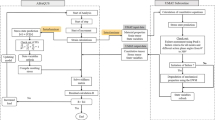
In this study, the material model described in the previous sections has been implemented using the subroutine code in finite element analysis ABAQUS software. In this process, the main problem is how to obtain the Jacobian matrix,$J = \partial \Delta {\sigma /}\partial \Delta {\upvarepsilon }$, where $\Delta {\upsigma }$ and $\Delta {\upvarepsilon }$ are the stress and strain increment tensors, respectively. Generally, for this purpose, the calculation of the strain rates in terms of the stress rates is needed. Also, according to Eq. (7), this needs that the damage rates D₂ and D₁₂ should be obtained. As can be seen from Eq. (17), the values of the mentioned parameter are nonlinear functions of the conjugate forces Y, which Jacobian matrix J must be obtained based on.

Therefore, in terms of the engineering elastic constants the inverse strain–stress relations in this case become as follows (O’higgins et al. 2005):

$$ \left[ {\begin{array}{*{20}c} {\dot{\varepsilon }_{11} } \\ {\dot{\varepsilon }_{22} } \\ {\dot{\gamma }_{12} } \\ \end{array} } \right] = \left[ {\begin{array}{*{20}c} {A_{11} } & {A_{12} } & {A_{13} } \\ {A_{21} } & {A_{22} } & {A_{23} } \\ {A_{31} } & {A_{32} } & {A_{33} } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\dot{\sigma }_{11} } \\ {\dot{\sigma }_{22} } \\ {\dot{\tau }_{12} } \\ \end{array} } \right] $$

(A1)

Inverting Eq. (A1):

$$ \left[ {\begin{array}{*{20}c} {\dot{\sigma }_{11} } \\ {\dot{\sigma }_{22} } \\ {\dot{\tau }_{12} } \\ \end{array} } \right] = \frac{1}{{A_{11} A_{22} A_{33} - A_{12} A_{21} A_{33} - A_{11} A_{23} A_{32} }}\left[ {\begin{array}{*{20}c} {A_{22} A_{33} - A_{23} A_{32} } & {A_{12} A_{33} } & {A_{12} A_{23} } \\ {A_{21} A_{33} } & {A_{11} A_{33} } & {A_{11} A_{23} } \\ {A_{21} A_{32} } & {A_{11} A_{32} } & {A_{11} A_{22} - A_{12} A_{21} } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\dot{\varepsilon }_{11} } \\ {\dot{\varepsilon }_{22} } \\ {\dot{\gamma }_{12} } \\ \end{array} } \right] $$

(A2)

or in incremental form:

$$ \left[ {\begin{array}{*{20}c} {\Delta \sigma_{11} } \\ {\Delta \sigma_{22} } \\ {\Delta \tau_{12} } \\ \end{array} } \right] = \frac{1}{{A_{11} A_{22} A_{33} - A_{12} A_{21} A_{33} - A_{11} A_{23} A_{32} }}\left[ {\begin{array}{*{20}c} {A_{22} A_{33} - A_{23} A_{32} } & {A_{12} A_{33} } & {A_{12} A_{23} } \\ {A_{21} A_{33} } & {A_{11} A_{33} } & {A_{11} A_{23} } \\ {A_{21} A_{32} } & {A_{11} A_{32} } & {A_{11} A_{22} - A_{12} A_{21} } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\Delta \varepsilon_{11} } \\ {\Delta \varepsilon_{22} } \\ {\Delta \gamma_{12} } \\ \end{array} } \right] $$

(A3)

Therefore, the Jacobian matrix is:

$$ J = \frac{1}{{A_{11} A_{22} A_{33} - A_{12} A_{21} A_{33} - A_{11} A_{23} A_{32} }}\left[ {\begin{array}{*{20}c} {A_{22} A_{33} - A_{23} A_{32} } & {A_{12} A_{33} } & {A_{12} A_{23} } \\ {A_{21} A_{33} } & {A_{11} A_{33} } & {A_{11} A_{23} } \\ {A_{21} A_{32} } & {A_{11} A_{32} } & {A_{11} A_{22} - A_{12} A_{21} } \\ \end{array} } \right] $$

(A4)

where A_ij, i, j = 1, 2, 3 is the material coefficient which will be calculated by substituting damage parameters [Eq. (17)] and their conjugate forces [Eq. (8)] in Eq. (7). These are given by (O’higgins et al. 2005):

$$ A_{11} = \frac{1}{{E_{11}^{0} }} $$

(A5)

$$ A_{12} = A_{21} = - \frac{{\nu_{12} }}{{E_{11}^{0} }} $$

(A6)

$$ A_{13} = A_{31} = 0 $$

(A7)

$$ \begin{gathered} A_{22} = - \frac{1}{{E_{22}^{0} \left( {1 - D_{2} } \right)}} \hfill \\ \quad + \frac{{\sigma_{22} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}\frac{{\frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}\frac{{b\frac{{\sigma_{22} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}}} + b\frac{{\sigma_{22} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}} - b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}\frac{{b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}}}}} \hfill \\ \end{gathered} $$

(A8)

$$ A_{23} = \frac{{\sigma_{22} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}\frac{{\frac{1}{{G_{12}^{0} \left( {1 - D_{12} } \right)}}\left( {\frac{{\sigma_{12} }}{{\left( {1 - D_{12} } \right)^{3} }} + \frac{{\sigma_{12}^{2} }}{{\left( {1 - D_{12} } \right)^{2} }}\frac{{\frac{{\sigma_{12} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }}}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}}}} \right)}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}} - b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}\frac{{b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}}}}} $$

(A9)

$$ A_{23} = \frac{{\sigma_{12} }}{{G_{12}^{0} \left( {1 - D_{2} } \right)^{2} }}\frac{{b\frac{1}{{E_{22}^{0} \left( {1 - D_{22} } \right)}}\left( {\frac{{\sigma_{22} }}{{\left( {1 - D_{22} } \right)}} + \frac{{\sigma_{22}^{2} }}{{\left( {1 - D_{12} } \right)^{2} }}\frac{{b\frac{{\sigma_{22} }}{{E_{22}^{0} \left( {1 - D_{12} } \right)^{2} }}}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}}}} \right)}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - \frac{{\sigma_{12}^{2} }}{{G_{22}^{0} \left( {1 - D_{12} } \right)^{3} }} - b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}\frac{{\frac{{\sigma_{12}^{2} }}{{G_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}}}}} $$

(A10)

$$ \begin{gathered} A_{22} = \frac{1}{{G_{12}^{0} }} \hfill \\ \, \left( {\frac{1}{{\left( {1 - D_{2} } \right)}} + \frac{{\sigma_{12} }}{{\left( {1 - D_{12} } \right)^{2} }}\frac{{\frac{{\sigma_{12} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{12} } \right)^{3} }}\frac{{\frac{{\sigma_{12} }}{{G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }}}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - b\frac{{\sigma_{12}^{2} }}{{E_{22}^{0} \left( {1 - D_{12} } \right)^{3} }}}}}}{{2Y_{12(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }}} - \frac{{\sigma_{12}^{2} }}{{E_{22}^{0} \left( {1 - D_{2} } \right)^{3} }} - b\frac{{\sigma_{22}^{2} }}{{E_{12}^{0} \left( {1 - D_{12} } \right)^{3} }}\frac{{\frac{{\sigma_{12}^{2} }}{{G_{12}^{0} \left( {1 - D_{2} } \right)^{3} }}}}{{2Y_{2(c)} \sqrt {\frac{{\sigma_{12}^{2} }}{{2G_{12}^{0} \left( {1 - D_{12} } \right)^{2} }} + b\frac{{\sigma_{22}^{2} }}{{2E_{22}^{0} \left( {1 - D_{2} } \right)^{2} }}} - b\frac{{\sigma_{22}^{2} }}{{E_{22}^{0} \left( {1 - D_{12} } \right)^{3} }}}}}}} \right) \hfill \\ \end{gathered} $$

(A11)